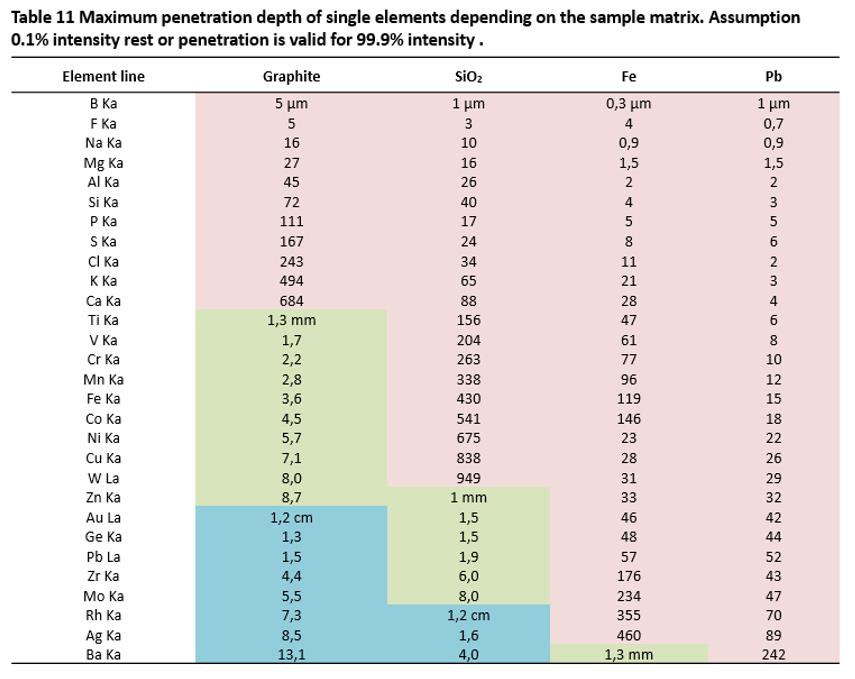
The fluorescence radiation for an element is absorbed as it leaves the sample. Beginning with a certain depth in the sample, the fluorescence signal is completely absorbed, i.e., all atoms that lie deeper than this can no longer be analyzed, as the radiation can no longer reach the surface. This depth is called the penetration depth or the infinite thickness.
The penetration depth increases with the atomic number. It is independent from the XRF instrument used, the excitation voltage or current from the X-ray tube. It is determined exclusively by the sample matrix.
Figure 34 clearly shows that an observed element is only analyzed in a layer up to the maximum penetration depth.
The energy of the observed element’s fluorescence radiation and the element in the surrounding sample matrix determine the maximum penetration depth.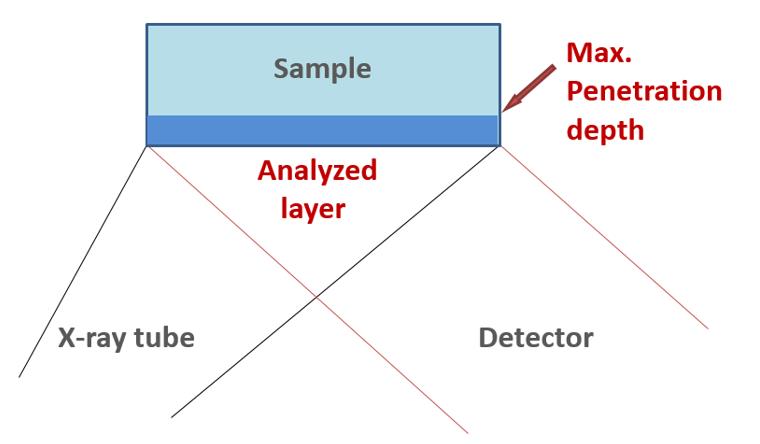
Formula
(15) can be used to calculate the penetration depth. It can be derived from
formulas (3) and (14). In formula (3), the linear absorption coefficient, µlin, is replaced by the mass
absorption coefficient, µ. In this way, the density, r, of the total sample is introduced into the
formula.
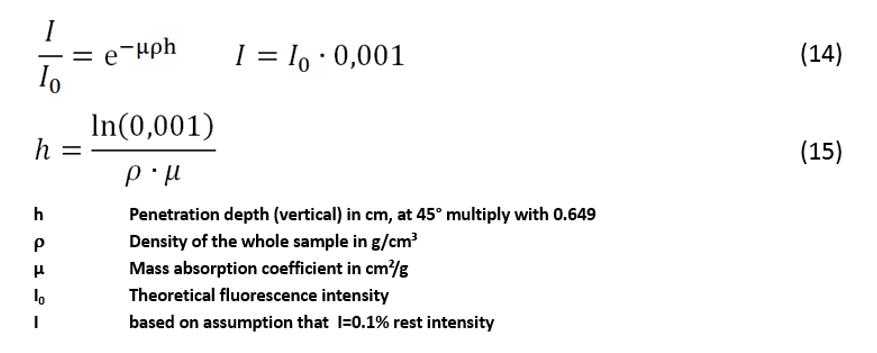
In
addition, it is assumed that after absorption along the distance, h, a residual intensity of 0.1% remains. The mass
absorption coefficients for every fluorescence line and for every pure element
matrix are listed in tables [23]. The
vertical penetration depths for a selection of elements in various matrices are
calculated in Table 11. The
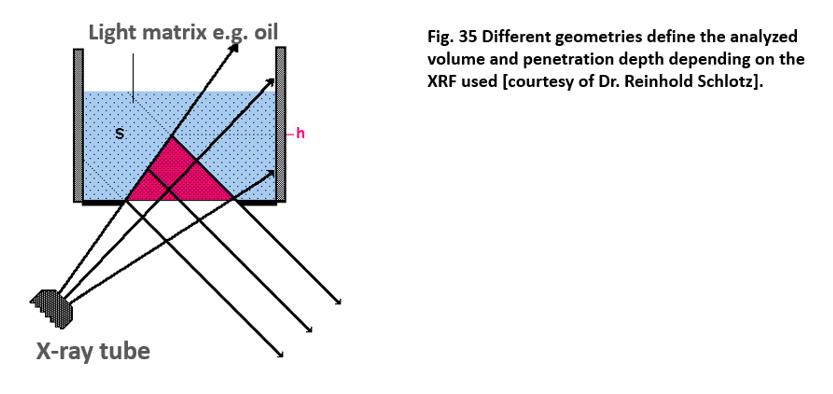
geometry of the excitation and the detection must be taken into consideration.
Because the detection is usually performed with a given angle (e.g., 45°), the
vertical penetration depth is reduced (Fig. 35). The fill
height plays a role in light matrices, such as oil. Even when it is higher than
the maximum penetration depth for the given element, a change can influence the
course of the background. One reason for this is that the excitation radiation
penetrates deeper due to its higher energy. Another reason is that more
multiple scattering occurs in light matrices.